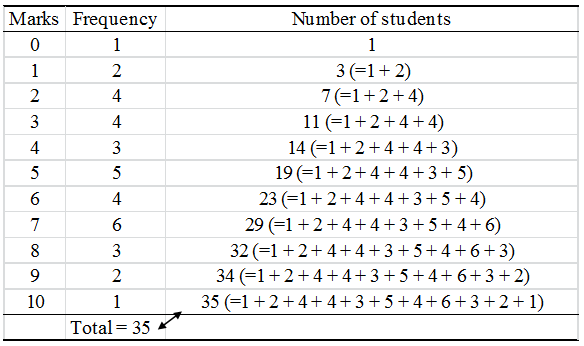
Cumulative frequency is defined as a running total of frequencies. The frequency of an element in a set refers to how many of that element there are in the set. Cumulative frequency can also defined as the sum of all previous frequencies up to the current point.
Example
Problem Statement:
The set of data below shows the ages of participants in a certain winter camp. Draw a cumulative frequency table for the data.
| Age (years) | Frequency |
| 10 | 3 |
| 11 | 18 |
| 12 | 13 |
| 13 | 12 |
| 14 | 7 |
| 15 | 27 |
Solution:
The cumulative frequency at a certain point is found by adding the frequency at the present point to the cumulative frequency of the previous point. The cumulative frequency for the first data point is the same as its frequency since there is no cumulative frequency before it.
| Age (years) | Frequency | Cumulative Frequency |
| 10 | 3 | 3 |
| 11 | 18 | 3 + 18 = 21 |
| 12 | 13 | 21 + 13 = 34 |
| 13 | 12 | 34 + 12 = 46 |
| 14 | 7 | 46 + 7 = 53 |
| 15 | 27 | 53 + 27 = 80 |