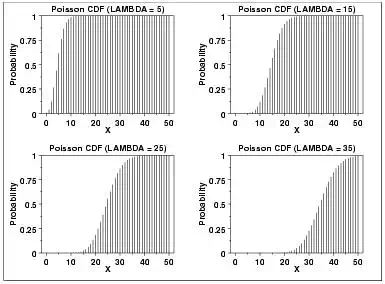
λλ is the shape parameter which indicates the average number of events in the given time interval. The following is the plot of the Poisson probability density function for four values of λλ. Cumulative Distribution Function.

Formula

F(x,λ)=∑k=0xe−λλxk!F(x,λ)=∑k=0xe−λλxk!

Where −

·        ee = The base of the natural logarithm equal to 2.71828

·        kk = The number of occurrences of an event; the probability of which is given by the function.

·        k!k! = The factorial of k

·        λλ = A positive real number, equal to the expected number of occurrences during the given interval

Example

Problem Statement:

A complex software system averages 7 errors per 5,000 lines of code. What is the probability of exactly 2 errors in 5,000 lines of randomly selected lines of code?

Solution:

The probability of exactly 2 errors in 5,000 lines of randomly selected lines of code is:

p(2,7)=e−7722!=0.022